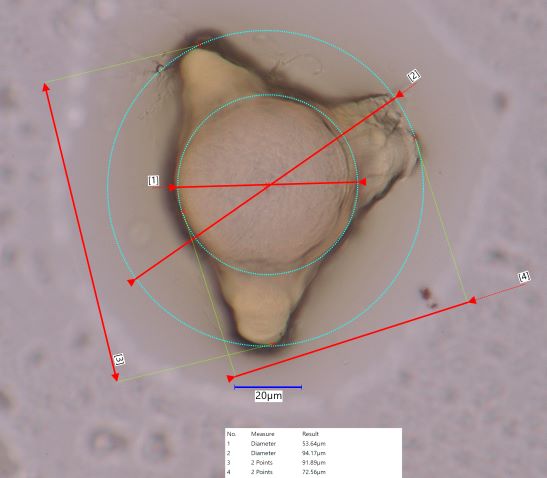
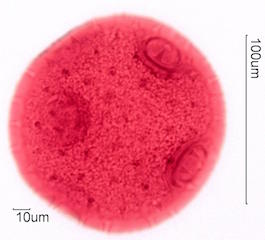
Size is a far more complex issue than might seem to be the case. In the fireweed pollen grain above, five different sizes are shown. The size of the largest enclosed circle is [1], 53.6um. The size of the smallest enclosing circle is [2], 94.2um. The longest dimension is [3], 91.9um. [4], 72.6um, is the Martin's diameter and one of the Ferret's diameters. There are many other sizes that could be calculated for this particle.

Size is simple for a circle, but quickly becomes more difficult as the aspect ratio increases or the length of the perimeter significantly exceeds the perimeter of the enclosed circle. There are a number of standard approaches. If bridging is a concern then the longest dimension of the particle is its size. If the concern is a sieve size then the larger of the two smallest dimensions is the size. The average of 6 or more Ferrets is another standard measure. Other measures include the diameter of the enclosing circle, the diameter of the enclosed circle, the projected intercept with a line at a fixed angle, aerodynamic equivalent diameter, diameter of a circle with the same area, diameter of a circle with the same perimter, and more.

Dimensions appear similiar

Particle Area = .25(pie)(d2) or 32.9um for the particle below.

Particle Perimeter = (pie)(d) or 43.9um for the particle below.

Equivalent Spherical Volume Diameter (30.43um) times (Equivalent Circular Area Diameter (32.9) divided by Equivalent Circular Perimeter Diameter (43.9)) or (30.43)(32.9/43.9) = 22.8um

The diameter of the largest enclosed circle. If the hieght above the slide is concidered the smallest diameter then two of the particle's dimensions are at or below this size. The two smallest dimensions determine size of the mesh that the particle will pass through.

The diameter of the smallest enclosing circle or longest dimension.

The Diameter of the Largest Enclosed Circle: Shortest Dimension.