|
Relief
Relief, simply speaking, is the contrast at the edge of a particle as a
result of differences in the refractive index between the particle
and the mounting medium. John Delly provides a more detailed definition in
his book, ESSENTIALS OF POLARIZED LIGHT MICROSCOPY AND ANCILLARY
TECHNIQUES. His definition is "Contrast between a specimen and its
surroundings (typically mounting media) due to the difference between
their refractive indices. The greater the numerical difference in refractive
indices, the stronger the relief. Expressed as positive or
negative; high, medium, or low."
Glass Fiber and Silica Phytoliths in 1.485 refractive index mounting
medium
The first image shows a glass fiber from an office ceiling acoustic tile. The
refractive index of the glass fiber is about 1.520.
The relief is moderate. The sign cannot be determined, positive or negative,
from this image. If it were negative, refractive index
lower than the mounting medium, then the fiber would become dark when the
stage is lowered (bright Becke line out). If it were positive,
refractive index higher than the mounting medium, then the fiber would
become bright when the stage is lowered (bright Becke line in).

This image shows a glass fiber (lowere left) and a silica phytolith (upper
right) with oblique illumination. The light is coming in
from the right. The silica phytolith has a lower refractive index, relief is
negative, and so is bright on the right. The glass fiber has a higher
refractive index, relief is positive, and is dark on the right side. With
oblique illumination the relative refractive index can be
determined without having to defocus the particle.

Calcite in 1.664
Calcite has an omega refractive index of 1.658 or higher (in this case 1.669)
and an epsilon prime refractive index in this orientation
of about 1.550. The first image shows the relief created when a linear
polarizer is oriented to show epsilon prime. The refractive index
difference is 0.114. The result is high relief. The second image is with the
crystal rotated 90 degrees, showing the omega refractive index.
The difference is 0.005 refractive index units. The result is low relief. In
this position, lowering the stage results in a orange Becke' line moving
into the particle and an blue Becke' line moving out, as shown in the third
image. If Oblique illumination is used the dispersion
staining colors are evident on oposite sides of the crystal.


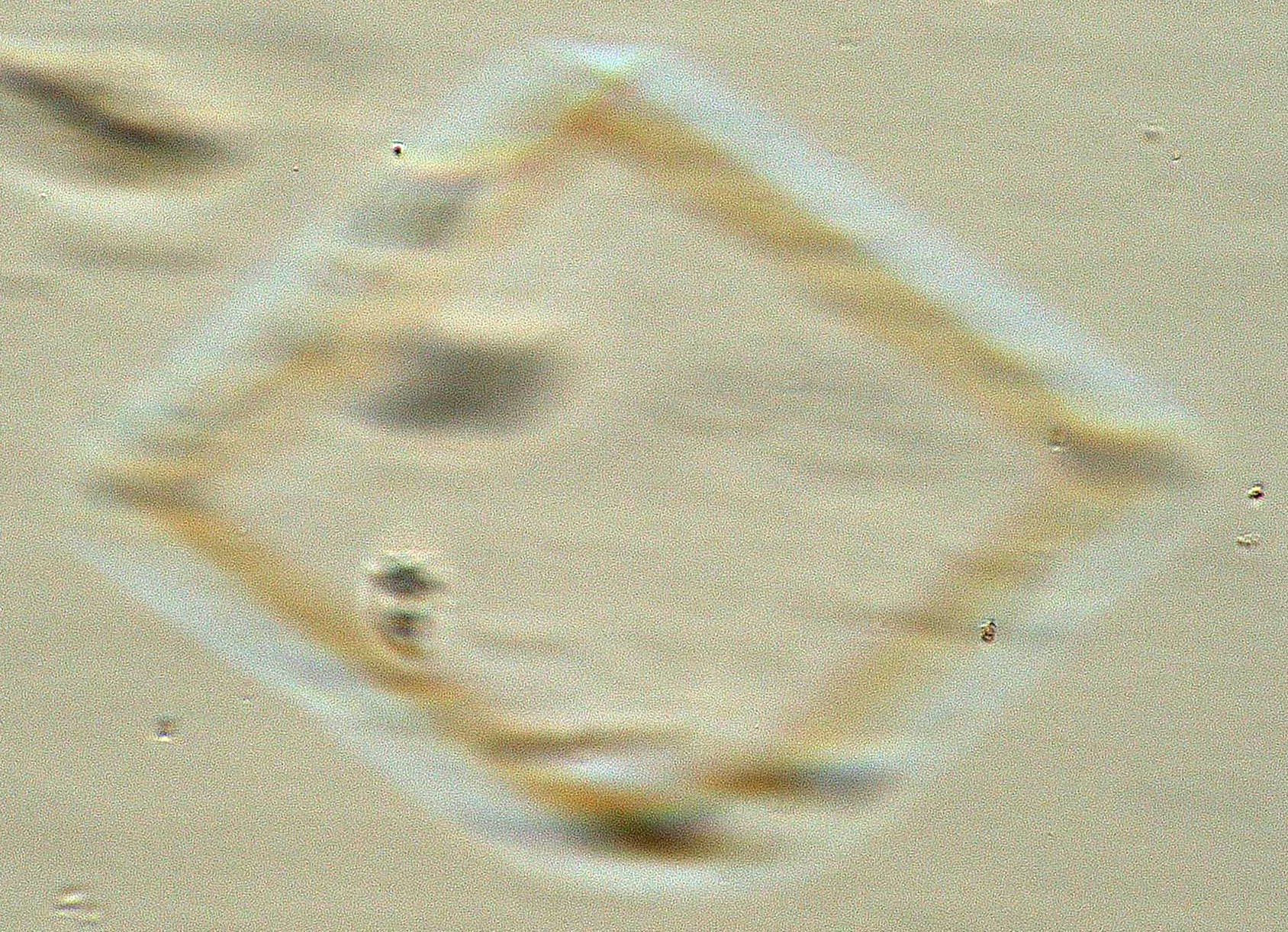
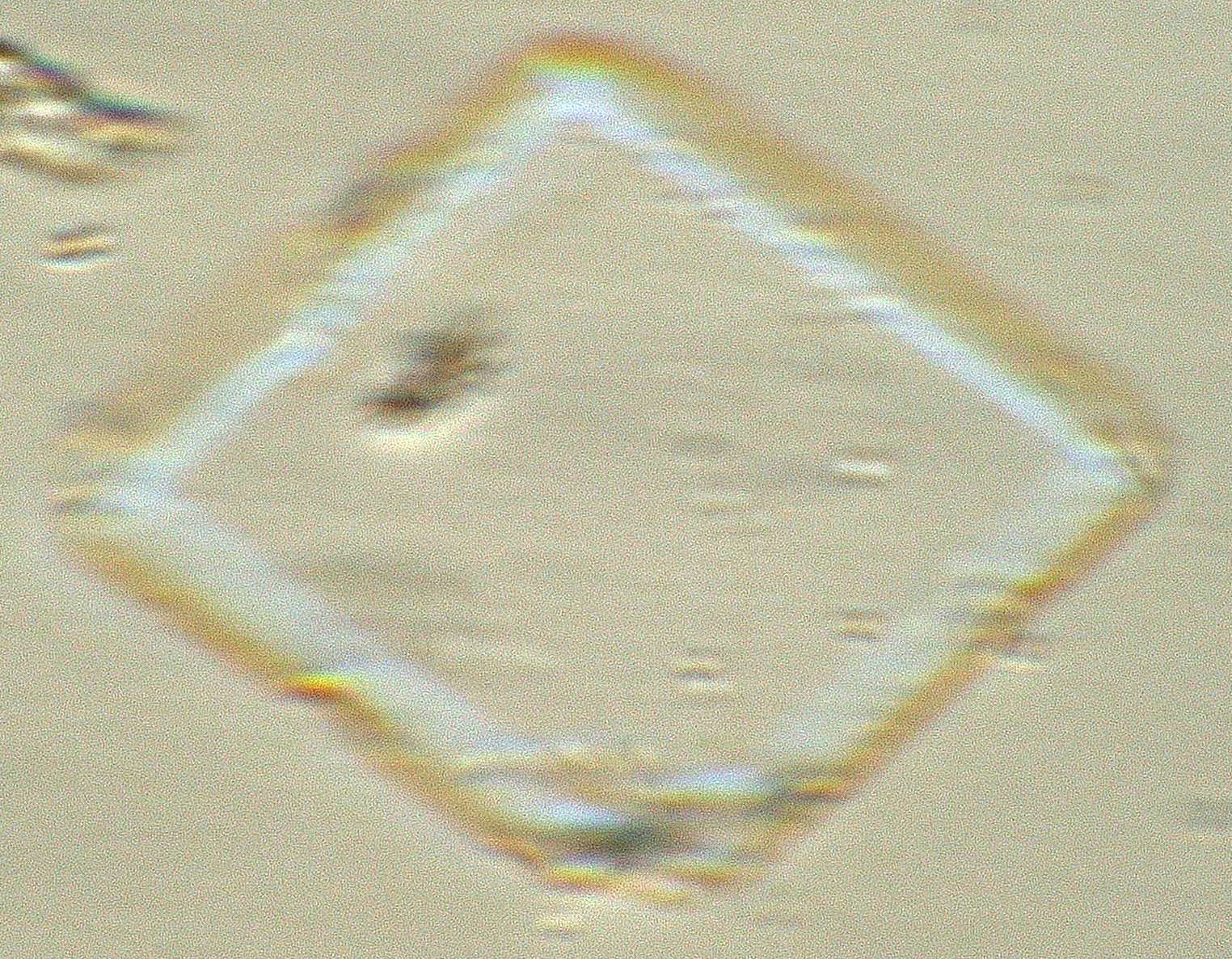
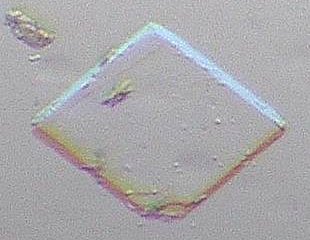
Calcite and Glass Standards in 1.664
The optical glasses have a refractive index of 1.64, 1.66, and 1.67. The
first image shows the calcite crystal in high relief and the
1.67 refractive index glass in low relief. The 1.66 refractive index glass
is invisible. With oblique illumination, the second image,
the optical glasses show dispersion staining colors.

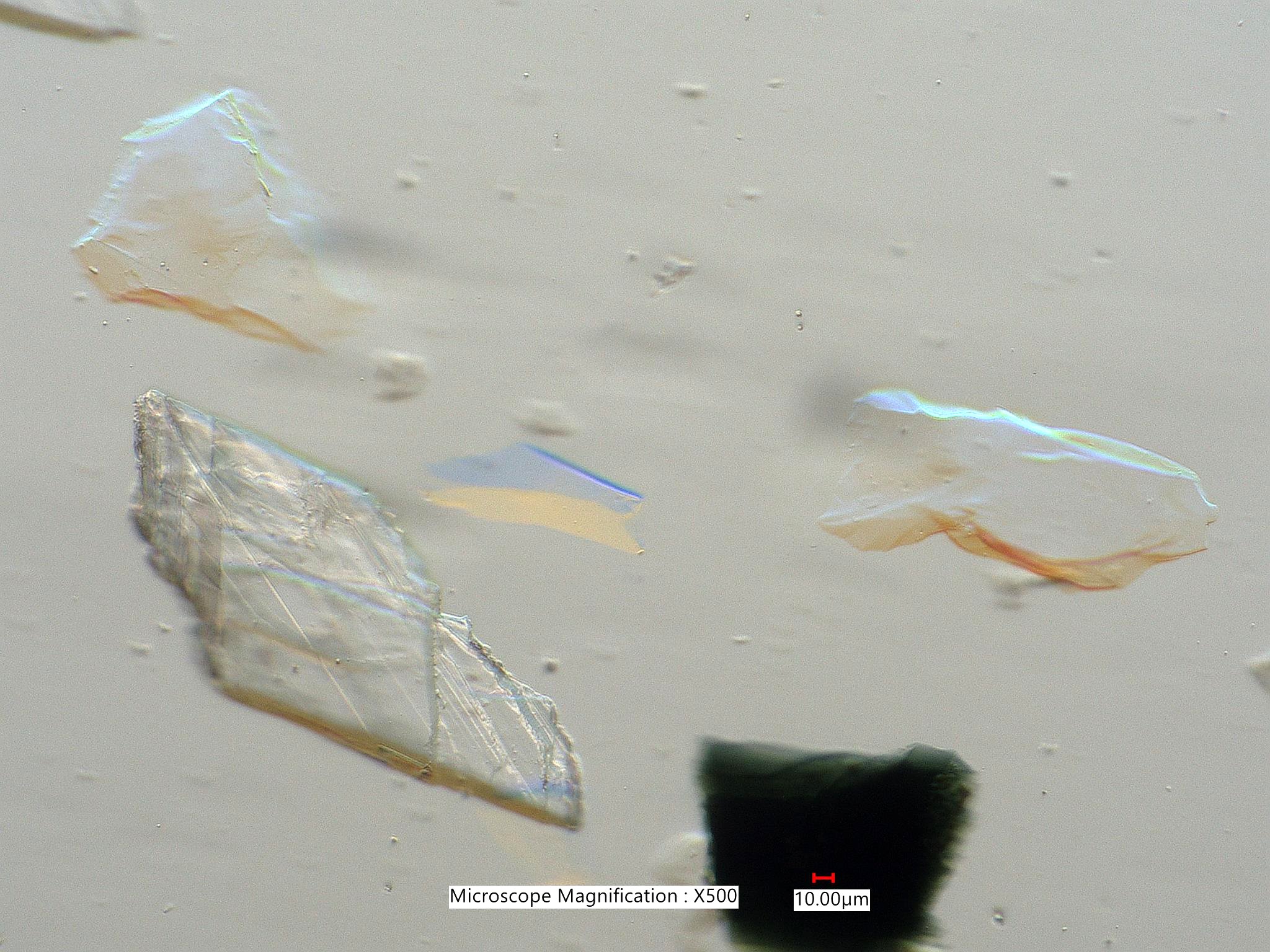
Olivine and Quartz in 1.664 Refractive index Medium
The first image shows the olivine (upper left) and quartz (lower center) in
slightly off crossed polarized light.
The second image is taken with oblique illumination and a single
linear polarizing filter oriented parallel to the high refractive
index of the olivine particle. The quartz is showing high negative relief.
It is bright on the side of the light source and dark on
the other side. That indicates that the refractive indices of the quartz
(1.544 and 1.553) are well below 1.664. The high refractive
index of the olivine particle is about 1.668, based on the dispersion colors
shown.
The third image shows the particles with the linear polarizer rotated
90 degrees. The relief of the quartz particle has not
changed. The olivine particle is now showing its low refractive index of
about 1.656. It is now showing low negative relief because
its refractive index in this position is slightly lower than the 1.664 of
the mounting medium.



Hornblend and Optical Glass Standards in 1.664 Refractive Index Medium

|